题目链接
https://leetcode.cn/problems/longest-substring-without-repeating-characters/
难度
中等
介绍
在上一篇易水介绍了自己对这个问题的解决方案,但那个方案执行效率和空间使用都很差。本文主要是介绍 stanislav-iablokov 的滑动窗口解决方案,他的算法时间复杂度为 O(n),空间复杂度为 O(1),完全可以用"简洁优雅"这四个字来形容!
C++解决方案
class Solution
{
public:
int lengthOfLongestSubstring(string s)
{
int max_len = 0;
// [1] longest substring is the one with the largest
// difference between positions of repeated characters;
// thus, we should create a storage for such positions
vector<int> pos(128,0);
// [2] while iterating through the string (i.e., moving
// the end of the sliding window), we should also
// update the start of the window
int start = 0;
for (int end = 0; end < s.size(); end++)
{
auto ch = s[end];
// [3] get the position for the start of sliding window
// with no other occurences of 'ch' in it
start = max(start, pos[ch]);
// [4] update maximum length
max_len = max(max_len, end-start+1);
// [5] set the position to be used in [3] on next iterations
pos[ch] = end + 1;
}
return max_len;
}
};算法分析
stanislav-iablokov的算法,思路正是上一篇中易水提过的想法:最长不重复子串,是两个重复字符之间的最大长度。但当时易水陷入了思维定势,始终想用字符查找、比较解决问题,但没有想到滑动窗口的解决方法。
在此算法中,stanislav-iablokov用 start 表示滑动窗口的起始位置,初始值为 0,后续循环中会将其值设为离起点最远的重复字符所在位置的后面(即其数组下标加1);end表示滑动窗口的结束位置,初始值为 0,在循环中依次后移;pos[128]数组用于存放每个字母上一次出现过的位置,初始值为0,代表字母没出现过。
在循环中,end会依次后移,然后会检查start是否需要后移:如果出现了重复字符,且重复字符在当前start之后,则将start后移至该重复字符的后面;否则start保持不变。在确定了滑动窗口的起始地址后,计算窗口中字符长度,即为不重复字符的长度,将其最大长度保存至 max_len 变量即可。
算法的精妙之处在于如何确定滑动窗口起始位置:如果在当前start之后存在end位置上的字符,说明在当前start和end间出现了重复字符,此时需要把start后移至重复字符的后面。移动之后,在start和end之间就不存在重复字符了。
另外,这个算法还打破了易水的思维定势,程序中完全没有用到字符查找、字符比较,而是用数组下标代之!
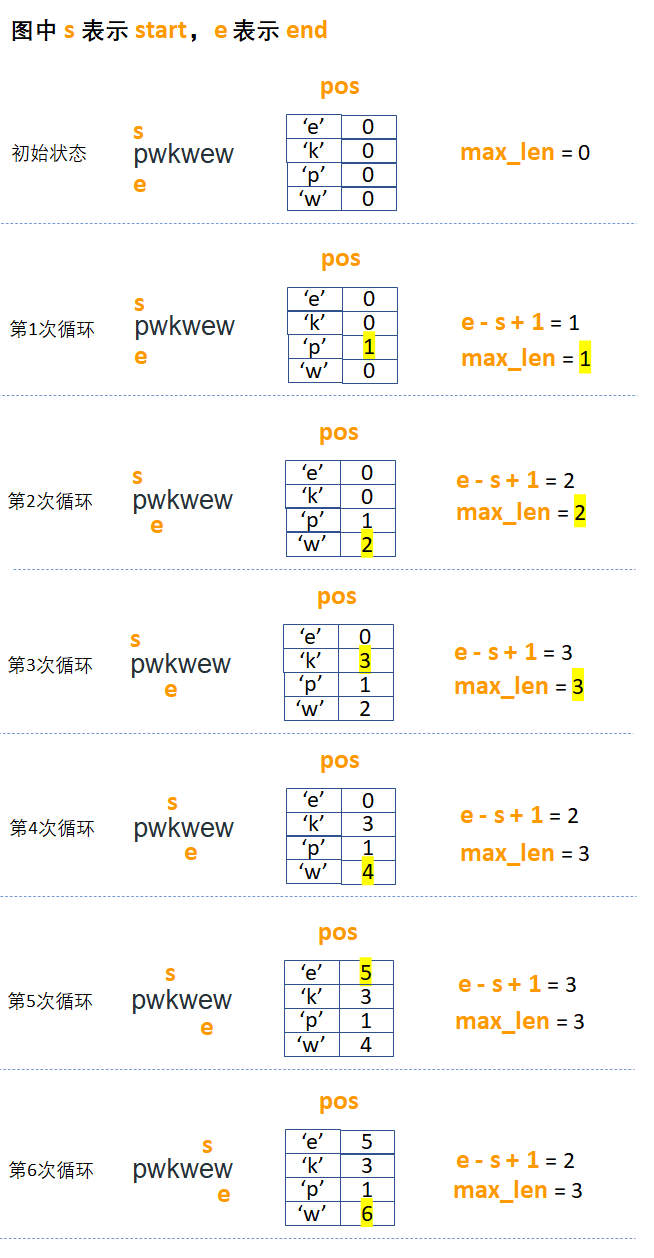
易水画了一副示意图,展示了该算法对于字符串 pwkwew 的处理过程:
总结
以后在遇到下面这类问题时,可以考虑滑动窗口方案:
- 给定一个序列,例如数组、字符串;
- 需要处理的数据是其中的一个子序列或子字符串;
- 给定了一个固定的窗口大小,或是要求找出窗口的大小(例如本题);
使用滑动窗口方案,关键在于窗口起点和终点的确定,这需要根据问题进行具体分析。
